Velocity of a wave on string

Since a string has elastic properties, the disturbance caused on a stretched string at a point, exerts force on neighbouring parts. Neighbouring part responds to this force and this response propagates on the string . Let 'F' be the elastic force in string.

Consider a wave travelling on the string be y = f (t – 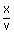). Let us choose an observer who is moving with speed 'V' in positive direction. For the observer the string does not move in y-direction as the disturbance is also travelling along with him, with the same speed. If a crest is opposite to him at an instant time(t), it will always remain opposite to him with the same shape while the string passes through this crest in opposite direction like a snake.

Consider a small element 'AB' of the string of length 'Δl'

θ1 ≅ θ2 = θ → 0
As the element 'AB' is very small θ1 ≅ θ2 = θ → 0
Resultant force in Radial direction = F sin θ1 + F sin θ2 ≅ 2F sin θ = 2F
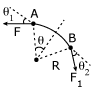
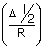
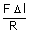FR = .... (i)

This radial force is causing the points on the string slide on the arc AB with speed 'V'.
Let the mass of an arc 'AB' be 'Δm'.

∴ FR = .... (ii)
From the equations (i) and (ii), we have
FR = =
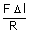⇒ v2 =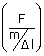
Let 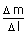= μ (linear mass density of string)
∴ v =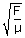