Theorems
Theorem - I:
Image

If P(x1, y1) is an external point to the ellipse S = 0, then the equation of the chord of contact to P is S1 = 0.

Proof:

Let the tangents through P(x1, y1) to the given ellipse S = 0 touch the ellipse at A(x2, y2) and B(x3, y3).

Then the tangent at A,

passes through P(x1, y1)

...... (1)

Similarly since P lies on the tangent at B is

...... (2)

From (1) and (2) points A(x2, y2), B(x3, y3) satisfy the equation S ≡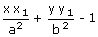 = 0

∴ S1 = 0 is the line representing AB, the chord of contact of P w.r.t. the given ellipse S = 0 [∵ S1 = 0, a linear equation, represents a line]
Theorem - II:

The polar of the point P(x1, y1) (other than the centre) w.r.t. ellipse S = 0 is S1 = 0.

Image
Proof:

Let Q and R be the points in which any chord drawn through the point (x1, y1) meets the ellipse S = 0.

Let the tangents at Q and R meet at the point M whose coordinates are (h, k)

∴ QR is the chord of contact of tangents from (h, k), its equation is and it passes through the point (x1, y1) we have

Since the relation (1) is true, it follows that the point (h, k) lies on the S1 ≡ = 0

Hence S1 = 0 is the polar of P(x1, y1) w.r.t. the ellipse S = 0.
Theorem - III:

The pole of the line lx + my + n = 0, (n ≠ 0) w.r.t. the ellipse S = 0 is .

Proof:

Let P(x1, y1) be the pole of the straight line

lx + my + n = 0 (n ≠ 0) ...... (1)
w.r.t. the ellipse S = 0
Then the polar of P(x1, y1) w.r.t. the ellipse is
S1 ≡ = 0 ...... (2)
Since the equation (1) and (2) represents same line, we have
⇒ x1 = , y1 =
∴ Pole of the line lx + my + n = 0, (n ≠ 0) w.r.t. the ellipse S = 0 is .
Theorem - IV:

If the polar of P w.r.t. S = 0 passes through Q, then the polar of Q w.r.t S = 0 passes through P.

Proof:

Let the coordinates of P and Q be (x1, y1) and (x2, y2) respectively and the equation of the ellipse be

S ≡ = 0
The polar of P w.r.t. S = 0 is S1 ≡ = 0. This passes through Q(x2, y2).
∴ S12 = = 0
The polar of Q(x2, y2) w.r.t. S = 0
i.e., = 0 passes through P(x1/>, y1).
Theorem - V:

The condition for two lines l1x + m1y + n1 = 0 and l2x + m2y + n2 = 0 (where n1 and n2 ≠ 0) to be conjugate w.r.t. ellipse S = 0 is a2l1l2 + b2m1m2 = n1n2.

Proof:

We have the pole of l1x + m1y + n1 = 0 w.r.t. the ellipse S ≡ = 0 is P

The given lines are conjugate
⇔ P lies on l2x + m2y + n2 = 0
⇔ l2 + m2 + n2 = 0
⇔ a2l1l2 + b2m1m2 = n1n2.