Find the area of a rectangle whose length and breadth are 45 cm and 18 cm respectively. Also, find the perimeter of the rectangle.
| Here, length = 45 cm and breadth = 18 cm. | ||
| Area of the rectangle | = | (length × breadth) sq. units |
| = | (45 × 18) cm2 | |
| = | 810 cm2 | |
| Perimeter of the rectangle | = | [2 × (length + breadth)] units |
| = | [2 × (45 + 18)] cm | |
| = | (2 × 63) cm | |
| = | 126 cm | |
Find the area and perimeter of a rectangular plot of land whose length and breadth are 28.5 m and 20 m respectively.
| Here, length = 28.5 m and breadth = 20 m. | ||
| ∴ Area of the plot | = | (length × breadth) sq. units |
| = | (28.5 × 20) m2 | |
| = |  m2 m2 |
|
| = | 570 m2 | |
| ∴ Perimeter of the plot | = | [2 × (length + breadth)] units |
| = | [2 × (28.5 + 20)] m | |
| = | (2 × 48.5) m | |
| = | 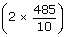 m m |
|
| = | 97 m | |
Find the cost of cultivating a rectangular field 80 m long and 35 m wide at Rs.15 per square metre. Also, find the cost of fencing the field at Rs. 24 per metre.
| Length = 80 m and breadth = 35 m. | ||
| Area of the field | = | (length × breadth) sq. units |
| = | (80 × 35) m2 | |
| = | 2800 m2 | |
| Rate of cultivation | = | Rs. 15 per m2 |
| ∴ Cost of cultivation | = | Rs. (2800 × 15) |
| = | Rs. 42000 | |
| Perimeter of the field | = | [2 × (length + breadth)] units |
| = | [2 × (80 + 35)] m | |
| = | (2 × 115) m | |
| = | 230 m | |
| Rate of fencing | = | Rs.24 per metre |
| ∴ Cost of fencing | = | Rs. (230 × 24) |
| = | Rs. 5520 | |
The area of a rectangle is 630 cm2 and its length is 35 cm. Find the perimeter of the rectangle.
| Area = 630 cm2 and length = 35 cm. | ||
| Breadth of the rectangle | = | 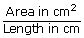 |
| = | 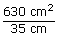 |
|
| = | 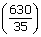 cm cm |
|
| = | 18 cm | |
| Perimeter of the rectangle | = | [2 × (length + breadth)] units |
| = | [2 × (35 + 18)] cm | |
| = | (2 × 53) cm | |
| = | 106 cm | |
| = | 1 m 6 cm | |
A room is 20 m long and 18 m broad. Find the cost of carpeting the room with a carpet of width 1.5 m at the rate of Rs. 60 per metre.
| Length of the room = 20 m and its breadth = 18 m. | ||
| Area of the floor of the room | = | (20 × 18) m2 |
| = | 360 m2 | |
| Area of the carpet required | = | 360 m2 |
| Width of the carpet | = | 1.5 m |
| Length of the carpet | = | 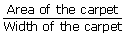 |
| = | 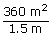 |
|
| = |  m m |
|
| = | 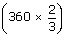 m m |
|
| = | 240 m | |
| Rate of carpeting | = | Rs. 60 per metre |
| Cost of carpeting | = | Rs. (240 × 60) |
| = | Rs. 14400 | |
The total cost of flooring a room at Rs. 85/m2 is Rs. 5100. If the length of the room is 8 metres, find its breadth.
| Total cost of flooring | = | Rs. 5100 |
| Rate of flooring | = | Rs. 85/m2 |
| Area of the floor | = |  |
| = | 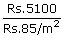 |
|
| = | 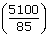 m2 m2 |
|
| = | 60 m2 | |
| Length of the floor | = | 8 m |
| ∴ Breadth of the floor | = | 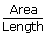 |
| = |  |
|
| = |  m m |
|
| = | 7.5 m | |
| Hence, the breadth of the floor is 7.5 m. | ||
A room is 8 m long and 6 m wide. Its floor is to be covered with rectangular tiles of size 25 cm by 20 cm. How many tiles will be required? Find the cost of these tiles at Rs. 25 per tile.
| Here, length of the room | = | 8 m and its width = 6 m. |
| ∴ Area of the floor | = | (8 × 6) m2 = 48 m2 |
| Area of each tile | = | 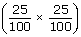 m2 = m2 =  m2 m2 |
| ∴ Number of tiles required | = | 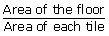 |
| = | 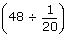 |
|
| = | (48 × 20) | |
| = | 960 | |
| ∴ Cost of tiles | = | Rs. (960 × 25) |
| = | Rs. 24000 |
How many square tiles each of side 0.5 m will be required to pave the floor of a room which is 4 m long and 3 m broad?
| Length of the room = 4 m and its breadth = 3 m. | ||
| Area of the floor of the room | = | (4 × 3) m2 |
| = | 12 m2 | |
| Each side of the square tile | = | 0.5 m =  m m |
| Area of each square tile | = | ( × ×  ) m2 ) m2 |
| = |  m2 m2 |
|
| Required number of tiles | = | (12 ÷  ) ) |
| = | (12 × 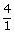 ) ) |
|
| = | 48 | |
| Hence, 48 tiles are required to pave the floor of the room. | ||