Heron's formula

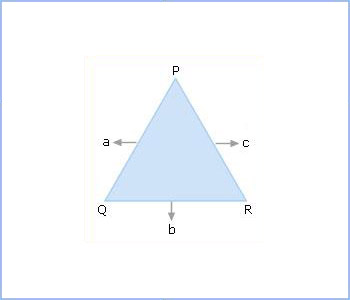
Let 'a', 'b', 'c' be the lengths of the sides of a Δ PQR.

Then, the area of Δ PQR is given as:

, where s = semi-perimeter of the triangle.

The above formula for calculating the area of triangle is known as Heron's formula.

Area of an equilateral triangle

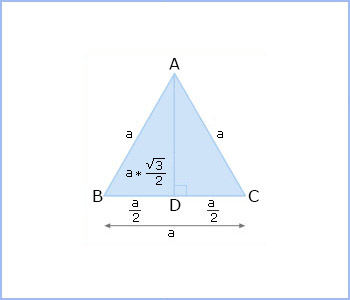
Let ABC be an equilateral triangle with side a.

Therefore, AB = BC = AC = a.

The height of an equilateral triangle is the length drawn from opposite vertex to that side.

Here, the height is given by AD =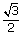

× a.

We know that, area of ^le	=	× base × height.
	=	× BC × AD
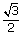	=	× a × a
	=
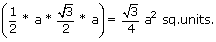Area of equilateral Δ^le with side 'a'	=

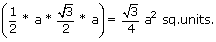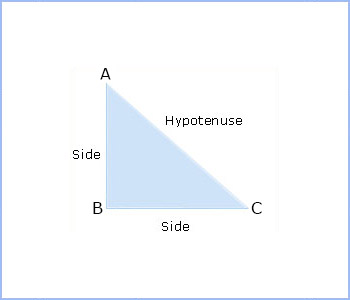
Area of a right-angled triangle

Let ABC be a right angled triangle with

B = 90°.

Area of Δ ABC =

× [Product of sides containing 90°]

=

× BC × AB sq. units.

Note: If ABC is a right angled triangle then Hypotenuse² = side² + side². If one side and hypotenuse is given, we can measure the other side and we can also measure the area.

Isosceles right-angled triangle

In a right angled triangle, if base = height then that becomes an isosceles right-angled triangle.

Let ABC be an isosceles right angled triangle. Therefore,

Area of Δ ABC	=	× [Product of sides containing 90°]
	=	× BC × AB.
	=	× AB × AB [BC = AB]
	=	× (AB)² sq. units.