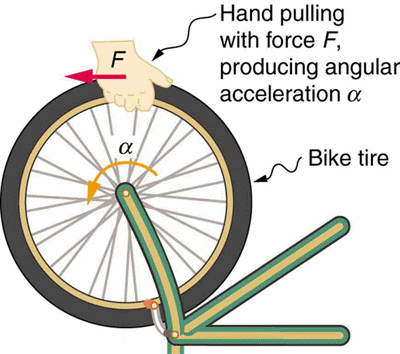
Demonstration of Newton's second law of rotation Analogy :
Force is required to spin the bike wheel. The greater the force, the greater the angular acceleration
produced. The more massive the wheel, the smaller the angular acceleration. If you push on a spoke closer to the axle, the angular
acceleration will be smaller.
The net force Σ F acting on an object of mass m determines the acceleration a that appears in the equations of linear kinematics.
Now, for a rigid body rotating about a fixed axis, we have seen that the rotational analog of Newton's law takes the form Σ τ = Iα .
A net torque Σ τ acting on an object that has a moment of inertia I causes an angular acceleration α. As is the case for linear motion, the angular acceleration provides the link between Newton's second law and the equations of rotational kinematics. If the net torque and the moment of inertia are known, the angular acceleration can be determined from the second law and then used in the equations of rotational kinematics. Conversely, if the angular acceleration can be found from the equations of rotational kinematics, it can then be used in Newton's second law to provide information about the net torque or the moment of inertia.
Basically it says that external Force acting with some momentum on the rotating body will change its angular
momentum, respective cause its angular acceleration. Newton's Second Law in translational motion looks like this:
force = (mass) × (acceleration)
The rotational analog to it is
torque = (moment of inertia) × (angular acceleration)