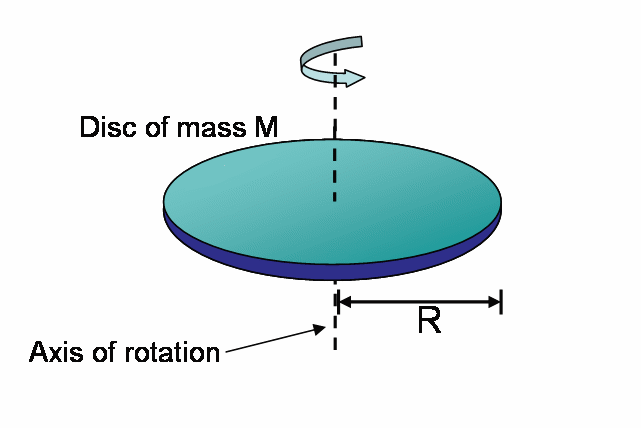
Moment of Inertia of disc of radius R and Mass ‘M’:
It is a measure of an object's resistance to changes to its rotation.
Also defined as the capacity of a cross–section to resist bending. It must be specified with respect to a chosen axis of rotation. It is usually quantified in kgm2. Moment of Inertia depends on the mass of the object, its shape and its relative point of rotation.
Moment of Inertia ‘I’ is a measure of an object's resistance to changes in rotation direction. Moment of Inertia has the same relationship to angular acceleration as mass has to linear acceleration. For a point mass the Moment of Inertia is the mass times the square of perpendicular distance to the rotation reference axis.
Mass is a measure of how difficult it is to get something to move in a straight line, or to change an object's straight–line motion. The more mass something has, the harder it is to start it moving, or to stop it once it starts. Similarly, the moment of inertia of an object is a measure of how difficult it is to start spinning, or to alter an object's spinning motion. The moment of inertia depends on the mass of an object, but it also depends on how that mass is distributed relative to the axis of rotation: an object where the mass is concentrated close to the axis of rotation is easier to spin than an object of identical mass with the mass concentrated far from the axis of rotation.
The moment of inertia of an object depends on where the axis of rotation is. The moment of inertia can be found by breaking up the object into little pieces, multiplying the mass of each little piece by the square of the distance it is from the axis of rotation, and adding all these products up.