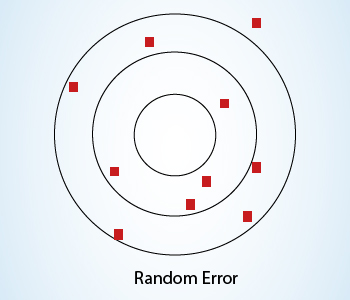
 Random error
Random error
All experimental measurements are subject to some uncertainty, or error. At the most fundamental level the uncertainty principle of physics tells us there are some things that we can never know exactly. But most measurements we are likely to make will be limited by ourselves or by our apparatus, and it is useful to learn to deal with these inherent shortcomings in our experiments. The process of collecting the data, finding out the errors and evaluating the results is collectively called as evaluation of data.
Classification of errors:
The errors observed in experiments are classified as determinate and indeterminate errors.
Indeterminate or random errors:
These are random in nature and lead to large variations in results. These cannot be corrected or eliminated. They can be
identified by repeated measurements of same variable.
Solved Example
The result of an analysis was determined as 15.952 g while the accepted value was 15.724 g. Find out the absolute error and the
relative error.
Solution:
| Absolute error | = | 15.952 − 15.724 |
| = | 0.228 g | |
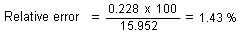 | ||
Absolute error:
The absolute error is the difference between the experimental value and the
true value (standard value). For example, if an analyst finds a value of 24.44% zinc in a sample which actually contains 24.35%
zinc, then the absolute error will be:
24.44 − 24.35 = 0.09%
Relative error:
It is the percentage of absolute error to the true value. In the above example, the relative error is
(0.09 × 100)/ 24.35 = 0.37%
or it may be expressed in parts per thousand i.e.,
(0.09 × 1000)/ 24.35 = 3.69 ppt (parts per thousand).