VSEPR theory assumes that electron groups minimize their repulsions and thus occupy as much space as possible around a central atom. But it does not explain how the shapes come from the interactions of atomic orbitals. After all, the orbitals we examined in earlier aren't oriented toward the corners of, for example, a tetrahedron or a trigonal bipyramid. Moreover, knowing the shape doesn’t help us to explain the magnetic and spectral properties of molecules; only an understanding of their orbitals and energy levels can do that.
Valence bond (VB) theory rationalizes observed molecular shapes through interactions of atomic orbitals that give rise to new “hybrid” orbitals. We apply VB theory to sigma and pi bonding, the two types of covalent bonds.
What is a covalent bond, and what characteristic gives it strength? And how can we explain molecular shapes based on the interactions of atomic orbitals? The most useful approach for answering these questions is based on quantum mechanics and calledvalence bond (VB) theory.
The basic principle of VB theory is that a covalent bond forms when orbitals of two atoms overlap and the overlap region, which is between the nuclei, is occupied by a pair of electrons. (“Orbital overlap” is another way of saying that the two wave functions are in phase, so the amplitude increases between the nuclei.) The central themes of VB theory derive from this principle.
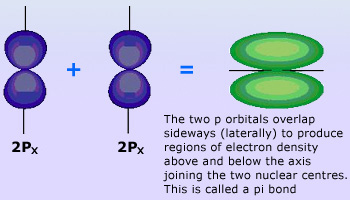Pi bond Showing overlapping of 2px and 2py Orbitals
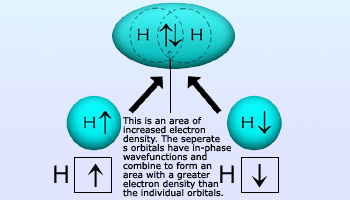Hydrogen molecule showing opposite spins of electron Pair
Opposing spins of the electron pair: As the exclusion principle prescribes, the space formed by the overlapping orbitals has a maximum capacity of two electrons that must have opposite spins. When a molecule of H2 forms, for instance, the two 1s electrons of two H atoms occupy the overlapping 1s orbitals and have opposite spins.
Maximum overlap of bonding orbitals: The bond strength depends on the attraction of the nuclei for the shared electrons, so the greater the orbitals overlap, the stronger (more stable) the bond. The extent of overlap depends on the shapes and directions of the orbitals. An ‘s’ orbital is spherical, but ‘p’ and ‘d’ orbitals have more electron density in one direction than in another. Thus, whenever possible, a bond involving ‘p’ or ‘d’ orbitals will be oriented in the direction that maximizes overlap. In the HF bond, for example, the 1s orbital of ‘H’ overlaps the half–filled 2p orbital of ‘F’ along the long axis of that orbital. Any other direction would result in less overlap and, thus, a weaker bond. Similarly, in the F–F bond of F2, the two half–filled 2p orbitals interact end to end, that is, along the long axes of the orbitals, to maximize overlap.
Examples Showing Overlapping Of Atomic Orbitals
Hybridization of atomic orbitals: To account for the bonding in simple diatomic molecules like HF, we picture the direct overlap of ‘s’ and ‘p’ orbitals of isolated atoms. But how can we account for the shapes of so many molecules and polyatomic ions through the overlap of spherical ‘s’ orbitals, dumbbell–shaped ‘p’ orbitals, and cloverleaf–shaped ‘d’ orbitals ?
To explain such facts, Linus Pauling proposed that the valence atomic orbitals in the molecule are different from those in the isolated atoms. Indeed, quantum mechanical calculations show that if we "mix" specific combinations of orbitals mathematically, we obtain new atomic orbitals. The spatial orientations of these new orbitals lead to more stable bonds and are consistent with observed molecular shapes. The process of orbital mixing is called hybridization, and the new atomic orbitals are called hybrid orbitals.
Applications of VB theory:
An important aspect of the VB theory is the condition of maximum overlap which leads to the formation of the strongest possible bonds. This theory is used to explain the covalent bond formation in many molecules.