| Substance | Sm(J K−1 mol−1 ) |
|---|---|
| Solids: | |
| Graphite, C(s) | 5.7 |
| Diamond, C(s) | 2.4 |
| Sucrose, C12H22O11(s) | 360.2 |
| Iodine, I2(s) | 116.1 |
| Liquids: | |
| Benzene, C6H6(l) | 172.3 |
| Water, H2O(l) | 69.9 |
| Mercury, Hg(l) | 76.0 |
| Gases: | |
| Methane, CH4(g) | 186.3 |
| Carbon dioxide, CO2(g) | 213.7 |
| Hydrogen, H2(g) | 130.7 |
| Helium, He(g) | 126.2 |
| Ammonia, NH3(g) | 192.4 |
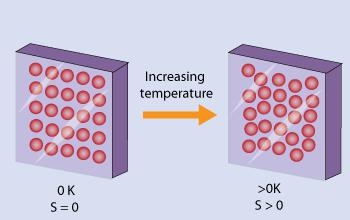
The 3rd law of thermodynamics will essentially allow us to make "sense" of entropies. It says that when we are considering a totally perfect (100% pure) crystalline structure, at a temperature of 0 kelvin (k), it will have no entropy (S). Note that if the structure in question were not totally crystalline, then although it would only have an extremely small disorder (entropy) in space, we could not precisely say it had no entropy. One more thing, we all know that at zero kelvin, there will still be some atomic motion present, but to continue making sense of this world, we have to assume that at absolute kelvin there is no entropy whatsoever.
The entropy change accompanying any physical or chemical transformation approaches zero as the temperature
approaches zero: S → 0 as T → 0 provided all the substances involved are perfectly ordered.
Entropy at an absolute temperature
First off, since absolute entropy depends on pressure we must define a standard pressure. It is conventional to choose the
standard pressure of just 1 bar. Also, from now on when you see "S" we mean the absolute molar entropy at one bar
of pressure. We know that ΔS = ST=final−ST=0; however, by the 3rd law this equation becomes
ΔS=ST=final.
Now note that we can calculate the absolute entropy simply by extrapolating (from the above graph) the heat capacities all the way down to zero kelvin. Actually, it is not exactly zero, but as close as we can possible get. For several reasons, it is so hard to measure the heat capacities at such low temperatures (T=0) that we must reserve to a different approach, much simpler. There is a law (Debye's 3rd thermodynamic law) that says that the heat capacities for most substances (doesn't apply to metals) is: C = bT3. It's possible to find the constant b if you fit Debye's equation to some experimental measurements of heat capacities extremely close to absolute kelvin. Just remember that b depends on the type of substance.
Finally, using Debye's law, we can calculate the molar entropy at values infinitely close to absolute kelvin temperatures: S (T) = (1/3)C(T) Note that C is the molar and constant volume heat capacity.