Antoine Lavoisier conducted many important studies on combustion reactions, considered as father of modern chemistry because of his carefully controlled experiment and his use of quantitative measurements. He was first to conclude that water is a compound of two gases Oxygen and Hydrogen.
Chemistry is a practical science. Just imagine how useful it could be to determine the formula of a compound from the masses of its elements or to predict the amounts of substances consumed and produced in a reaction.
Suppose you are a polymer chemist preparing a new plastic: how much of new material will a given polymerization reaction yield? Or suppose you're a chemical engineer studying rocket engine thrust: what amount of exhaust gases will a test of this mixture fuel mixture produce? Perhaps you are on a team of environmental chemists examining coal samples: what quantity of air pollutants will this sample release when burned? Or, may be you are a biomedical researcher who has extracted a new cancer preventing substance from a tropical plant: what is its formula, and what quantity of metabolic products will establish a safe dosage level? You can answer countless questions like these with the knowledge of stoichiometry. Conversion Calculations using the Dimensional Analysis method.
12 pieces make a dozen of eggs, making 12 a conversion factor for dozen
Defining Conversion factors:
A conversion factor is derived from a defined relationship between two sets of units.
The conversion factor above was obtained from the definition of 1 yard:
1 yard = 36 inches
Two conversion factors can be obtained from every defined equality. For example,
dividing both sides of the above equivalence by 1 yard gives
1 yard / 1 yard = 36 inches / 1 yard = 1
Dividing both sides by 36 inches gives
1 yard / 36 inches = 36 inches / 36 inches = 1
How do you use Conversion Factors? We all know from elementary school math that
if you multiply any quantity by 1 you get the same quantity back. You can do this
as many times as you want.
For example, 2 × 1 = 2, and 18 × 1 × 1 × 1 = 18.
Multiplication by 1 is what you do whenever you do a problem involving conversion
factors. The best way to explain how to solve using conversion factors is to work
through some simple examples.
Conversion of Metric units:
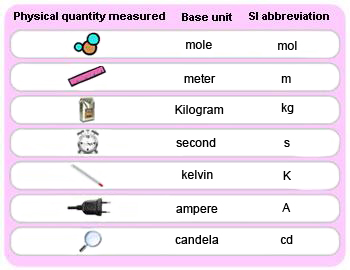
System International or S.I system is the modern version of metric system.
Seven Base S.I. Units
Conversion factors between a unit with a prefix and the corresponding metric base unit may be quickly obtained by first writing equality:
1 cm = 1 cm
and then replacing one of the prefixes with the corresponding exponent:
1 cm = 1 × 10−2 m
This equality can be used to write the two possible conversion factors as (1 cm / 10−2m) and (10−2m / 1 cm). Conversion between a prefix and a base unit is a one–step calculation, but conversion between two different prefixes requires two steps.
Worked out example
Convert 4.25 cm to meters and millimeters.
Sol: For the first conversion one of the conversion factors above may be used:
m = 4.25 cm (setup)
= 4.25 cm (10−2 m / 1 cm)
= 0.0425 m.
For the second conversion, centimeters to millimeters, two conversion factors are needed. The first converts the prefix to the base unit, and the second converts the base unit to the new prefix.
? mm = 4.25 cm (set up)
= 4.25 cm ( 10−2 m / cm ) (mm / 10−3 m )
= 42.5 mm.
The answers to both parts of this exercise were written to exponential notation in order to show that the number 4.25 do not change in these conversions, but the exponent does.
Conversion of Complex units:
The data used in chemistry involve complex units. There are area measurements that have squared units such as square kilometres (km2), square meters (m2), or square centimeters (cm2), cubic millimeters (mm3), and cubic meters (m3). For velocity the units may be meters per second, which is abbreviated as m/s or ms−1. Acceleration has units of meters per second squared (ms−2) and energy has units of kilogram meters squared per second squared (kg m2s−2).